The Cubic Conundrum

The quest for a universal method to solve cubic equations dates back centuries, with ancient civilizations such as the Babylonians, Greeks, and Persians encountering significant obstacles. Luca Pacioli, who taught mathematics to Leonardo da Vinci, famously asserted the unsolvability of cubic equations, a view shared by many across ancient cultures.

Historically, mathematicians approached equations with a geometric perspective, visualizing shapes and areas. While today's methods for solving quadratic equations are well-known, negative solutions were often ignored due to their real-world implications concerning lengths and volumes. This aversion to negative numbers resulted in multiple versions of quadratic and cubic equations.

In 16th-century Italy, Scipione del Ferro discovered the secrets of cubic equations but kept his findings a secret for job security. After his death, his student Antonio Fior revealed the solution, resulting in a mathematical rivalry. Del Ferro’s method faced its first public challenge, and Fior’s arrogance ultimately led to his downfall.

Niccolo Fontana Tartaglia, a self-taught mathematician who overcame a stutter, accepted Fior’s challenge. With a mix of skepticism and determination, Tartaglia not only solved Fior’s complex cubics but also introduced a revolutionary geometric approach. He expanded the idea of completing the square into three dimensions, systematically addressing depressed cubic equations through innovative volume breakdowns. This geometric breakthrough marked a significant advancement in the ongoing battle against cubic equations.

The Transition from Cubic Equations to Complex Numbers

Tartaglia’s innovative formula laid the groundwork for further exploration. Cardano, a physician and scholar, aspired to publish this solution but felt bound by his promise to Tartaglia. A serendipitous discovery in Bologna revealed a solution that predated Tartaglia’s work, allowing Cardano to publish "Ars Magna," which explored geometric proofs for cubic equations.

"Ars Magna" acknowledged various contributions but also stirred controversy. Some cubic equations, such as x³ = 15x + 4, presented challenges that introduced square roots of negative numbers. Despite initial hesitations, Cardano’s methodology prevailed.

Rafael Bombelli tackled the perplexity surrounding square roots of negatives by proposing a new type of number. By treating the square root of negative one as a separate entity, Bombelli successfully integrated it into Cardano’s methods, unveiling the profound potential of complex numbers.

The 1600s marked a pivotal shift from geometric reasoning to symbolic algebra. Francois Viete introduced modern algebraic notation, while Descartes popularized the term "imaginary numbers." The cubic dilemma initiated a separation of algebra from geometric constraints.

In the 20th century, Schrödinger's exploration of wave equations revealed the critical role of imaginary numbers in physics. The Schrödinger equation, incorporating i (the square root of negative one), became fundamental in explaining atomic behavior, bridging chemistry and physics.

The Unseen Power of Imaginary Numbers

Imaginary numbers reside on a perpendicular dimension to the real number line, forming what is known as the complex plane. When multiplied by i, they enact a 90-degree rotation within this plane—an essential characteristic for describing waves.

The first video titled "Determine a Real, Imaginary, and Complex Number" explores the foundational concepts of these number types, illustrating their definitions and applications in mathematics.

In 1926, Erwin Schrödinger presented his groundbreaking wave equation, which transformed the mathematical understanding of quantum particles. His approach diverged from classical physics, which primarily relied on real numbers. By incorporating complex numbers and the imaginary unit 'i' to denote the square root of -1, Schrödinger shifted the paradigm of classical mechanics and ignited debates among physicists.

Initially, his use of complex numbers met with skepticism from some contemporaries. However, Schrödinger's formalism yielded precise predictions, successfully elucidating the behavior of electrons within atoms, thereby gaining acceptance despite initial reservations.

The Schrödinger wave equation is represented as follows:

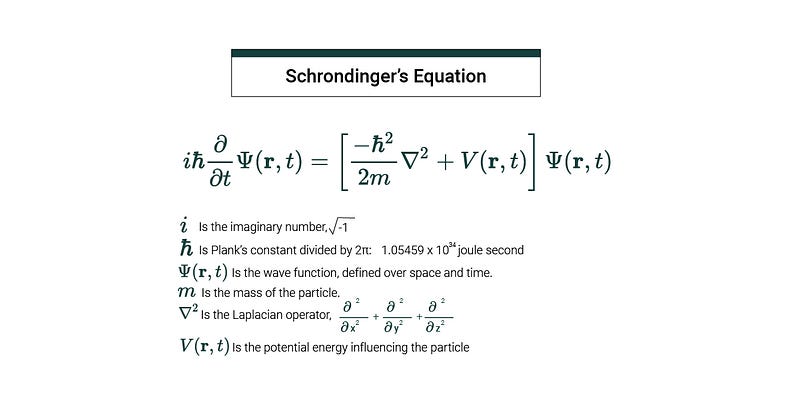
In this equation, ? signifies the wave function, ? is the reduced Planck constant, t denotes time, m represents the particle's mass, ?^2 is the Laplace operator, and V indicates potential energy. A defining feature of Schrödinger’s formulation is the introduction of complex numbers, particularly the imaginary unit i (where i^2 = -1). This unconventional application in physics provoked skepticism and discussions among physicists.

Prominent physicist Freeman Dyson (not to be confused with the vacuum cleaner brand) emphasized the unexpected importance of imaginary numbers. He argued that the mathematical framework of quantum mechanics, enhanced by complex numbers, offered a more accurate representation of physical reality. The inherently complex wave functions allowed for a probabilistic interpretation of particle behavior, challenging traditional deterministic views.

Over time, the significance and necessity of complex numbers in quantum mechanics became increasingly evident. They became an indispensable tool for physicists, providing a unique perspective to interpret the intricacies of the quantum realm.

The second video, "Imaginary Numbers Are Real [Part 1: Introduction]," delves into the fascinating world of imaginary numbers, discussing their historical context and significance in mathematics and physics.